LII:Basic Physics of Nuclear Medicine/MRI & Nuclear Medicine

This is a developing chapter of a wikibook entitled Basic Physics of Nuclear Medicine.

Magnetic resonance imaging (MRI) is widely used to provide colocalization information for correlative applications with nuclear medicine images. An introduction to this imaging method is provided in this chapter. We'll consider fundamental features to a depth sufficient to describe the contrast and spatial characteristics of MR image data so that spatial correspondence features with nuclear medicine data can be appreciated. Since MRI is such a vast field, we'll limit our treatment to a description of essential features only and you should refer to the Bibliography for more comprehensive accounts.

We'll start with an overview of the imaging process and then describe magnetic resonance features of the simplest atomic nucleus known, i.e. ¹H. From there we'll describe phenomena which generate contrast in MR images and finish with a description of image formation using spatial encoding techniques.

MR Imaging Process

In principle, MRI is quite a simple imaging technique, as illustrated in the animated graphic below. A patient is first of all placed inside a large magnet – the poles of which are indicated by the N and S boxes in the figure. Radio waves are then used to excite the nuclei of hydrogen atoms within the patient's body using an external coil and these are subsequently re-emitted by the patient in a manner which is characteristic of the NMR properties of the tissues involved. These radio waves are detected using an external coil, digitized, processed by a computer and displayed as tomographic slices revealing the distribution of different tissues.

Representation of the water molecule with the oxygen atom in blue and the two hydrogen atoms in red. The human body contains a substantial amount of water and therefore has a large number of hydrogen nuclei distributed throughout its volume to generate NMR signals for imaging purposes.

The imaging process is a bit more complex in practice, as indicated in the following figure:

Flow diagram for the MR imaging process.

Illustration of the principal components of MRI scanner. Tx and Rx refer to RF transmitter and receiver circuitry, respectively. The gradient coils are used to establish gradients in the magnetic field generated by the magnet.

The complexity arises from the use of gradient magnetic fields and sophisticated radio wave excitations so as to provide spatial localization information for the subsequent detection phase, and to isolate a particular NMR characteristic of interest. One powerful feature of MRI is that the method can generate images depicting a number of different contrast characteristics. Three such image types are shown as examples below:

Three types of MR image: the T1 weighted image depicts relatively bright grey matter and dark CSF; the T2 weighted image highlights the CSF, while the PD weighted image shows little contrast between tissues.

The physics behind the production of these types of images is briefly described in this chapter. We'll start with some insights into the nature of nucleons which we have not considered previously in this wikibook.

#top

Nuclear Spin

Our earlier consideration of the atomic nucleus concentrated on its composition in terms of the mass, electrical charge and the relative number of its neutrons and protons – the intention being to introduce the phenomenon of radioactivity. We neglected to mention, for the sake of simplicity, that nucleons can be considered to spin in space around a central axis. We'll now concentrate on this spinning characteristic in order to introduce the phenomenon of nuclear magnetic resonance (NMR), and we'll see later how images can be formed on this basis.

Let's take an isolated proton as an example – see the following figure:

A spinning proton produces a magnetic field similar to a bar magnet.

Besides having an electrical charge, the proton also spins on its axis. Since this spinning constitutes a moving electrical charge (i.e. rotating around a central axis), a magnetic field is generated, as illustrated, similar to that generated by a bar magnet – but on a much smaller scale, of course. This spinning magnet aligns itself with any external magnetic field.

There's an additional feature to this rotation, however, called precession, which can be seen in many rotating objects, as illustrated by the spinning gyroscope below:

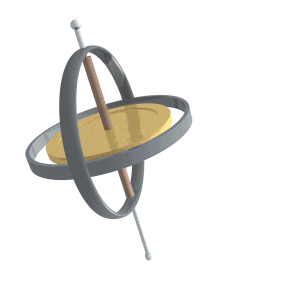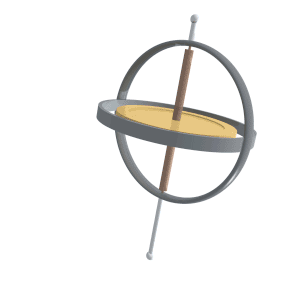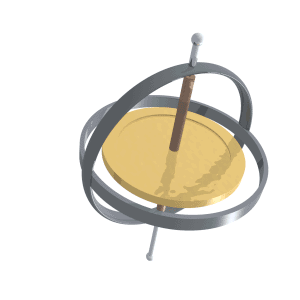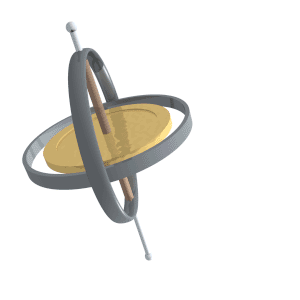
The rate of proton precession is what's important in NMR and has an angular frequency referred to as the Larmor frequency. Its specified by the gyromagnetic ratio of the nucleus in question and for hydrogen it has a value of 2.68x10⁸ rad/s/T. This ratio implies a rate of 42.58 million precessions per second when a hydrogen nucleus is placed in a magnetic field of strength 1 Tesla, i.e. rather higher than that for the precession shown in the animation above!

The following equation is of relevance here:

f = γ B_o

where f is the precessional frequency, γ is the gyromagnetic ratio and B_o is the strength of the magnetic field. This is a very important relationship in NMR and is exploited in numerous ways in MRI, as you will see below.

Clinical MRI scanners typically use field strengths between 0.1 and 3 T depending on the application. The strength of the earth's magnetic field in comparison is about 50 μT, i.e. about 2,000 to 60,000 times smaller, while that of a magnetic door lock is about 0.1 T.

We've just considered the situation for an isolated proton in a magnetic field. An interesting phenomenon occurs when many billions of protons, in a sample of tissue for example, are placed in a magnet:

some of the protons line up in parallel with the field and some line up against it.

These two states are referred to as parallel and anti-parallel states, as illustrated in the following figure:

The protons align themselves either parallel or anti-parallel to an external magnetic field, B_o. The anti-parallel state is slightly lower in energy than the parallel state. Transitions from the lower energy to the higher energy state can occur if an exact amount of energy ΔE is supplied.

The above figure also indicates that the two alignments are equivalent to two quantum energy states separated by an energy difference, ΔE. More nuclei occupy the lower energy than the higher energy state, but their excess is incredibly small – less than 0.001%. Its enough, however, to exploit in NMR!

This energy difference is directly related to the precessional frequency through the following equation:

ΔE = h f

where h is Planck's Constant and f is the precessional frequency, related to the Larmor frequency, ω, through:

ω = 2 π f

The important point here is that:

transitions from the parallel to the anti-parallel state can be induced in the sample when it is excited with electromagnetic radiation of energy, ΔE.

This energy is about 1.75x10^-7 eV for a proton in a 1 T field, i.e. a tiny amount of energy compared with electron binding energies, for instance. Compared to a 140 keV gamma-ray emitted by ^99mTc, its energy is over a million, million times smaller.

The energy difference is equivalent to the energy of electromagnetic radiation in the radio frequency (RF) region of the electromagnetic spectrum, as illustrated below:

The electromagnetic spectrum indicating regions exploited in nuclear medicine, radiology and MRI.

So, if we had a tissue sample placed in a magnetic field of 1 T and we beamed in radio waves at 42.58 MHz, we can expect to excite protons from the parallel to the anti-parallel state. This phenomenon is the resonance feature of NMR.

Its what happens following excitation that's exploited in MRI:

transitions back to the parallel state occur spontaneously over a time period which is characteristic of individual tissues and their various pathological conditions.

These transitions cause electromagnetic radiation to be emitted from our tissue sample whose amplitude decreases with time. The detected emissions form what is called a Free Induction Decay, as illustrated below:

A free induction decay characterized by a time constant, T2*.

Its the time constant of signal decays such as these which is determined in MRI and which can be encoded into MR images – once we've figured out where within the patient the signals have originated from.

#top

Magnetic Sum Vector

Its useful before proceeding to consider the situation from a magnetic vector perspective, as illustrated in the following figure:

Illustration of the cancelling effect feature of parallel and anti-parallel vectors.

The figure shows the situation for a hypothetical tissue sample placed in a magnetic field such that seven hydrogen nuclei are in the parallel state and five are in the anti-parallel state. Five of the parallel vectors cancel the effects of the five anti-parallel vectors and we're left with a net condition of two parallel vectors. We can simplify the situation further by considering the summation of these two vectors to give us a magnetic sum vector along the Z-axis, as illustrated in the following diagram:

Illustration of the magnetic sum vector, M_z.

Since the magnetic field direction is generally along the Z-axis in MRI, our magnetic sum vector is referred to as Longitudinal Magnetization. As a result, a longitudinal magnetization becomes established when a patient is placed inside the magnet of an MRI scanner.

#top

RF Excitation

Let's consider the situation once longitudinal magnetization has become established and when a pulse of RF radiation at the resonant frequency is beamed into our tissue sample, as illustrated in the following diagram:

Two processes occur simultaneously during absorption of the radiant energy:

Reduction in M_z: Some of the protons resonate and move to the anti-parallel state, resulting in a reduction in the longitudinal magnetization, as illustrated below:

Illustration of the reduction in M_z during RF excitation.

Notice that our initial situation with five protons aligned parallel, which gives rise to a longitudinal magnetization, M_z, of relatively large magnitude, changes to one where three are left with this alignment and two with the anti-parallel alignment. The magnitude of M_z is reduced as a result.

Phase Coherence: the vectors align with each other in phase, as illustrated below:

Illustration of the establishment of transverse magnetization.

The outcome of phase coherence is the establishment of a magnetic sum vector in the X-Y plane, called the Transverse Magnetization.

So, once longitudinal magnetization is established by placing a patient in the magnet of an MRI scanner and radio waves at the resonant frequency are generated, we in effect establish a transverse magnetization while reducing the longitudinal magnetization. Its what happens next to these two magnetic sum vectors, M_z and M_xy, that's of interest in MRI.

#top

RF Emission

We can consider the emission phase of the MR imaging process from both the longitudinal as well as the transverse magnetization perspectives. Since the protons in our tissue sample have been excited by the absorption of RF energy at the resonant frequency, the emission phase corresponds with what is called relaxation as protons return to their lower energy state.

Longitudinal Relaxation

The situation following excitation is illustrated in the top left panel of the following figure:

Illustration of longitudinal relaxation.

A hypothetical situation is shown where four protons are aligned parallel to the external magnetic field, and four excited protons are aligned anti-parallel. The magnetic sum vector is zero at this stage. Excited protons then return progressively to their lower energy state, as shown by the other panels in the above figure. The result is a re-establishment of the longitudinal magnetization, M_z, as illustrated in the following figure:

It is seen in this figure that the longitudinal magnetization becomes re-established in an exponential manner characterized by a time constant, called T1.

Transverse Relaxation

The situation following excitation is illustrated in the top left panel of the following figure, with phase coherence taken into account. As a result, a transverse magnetization, M_xy becomes established:

A hypothetical situation is illustrated where three protons are aligned parallel and three anti-parallel, in phase coherence with each other. The other panels in the figure illustrate a progressive loss of coherence that is characterized by this form of relaxation, where the transverse magnetization decreases to zero, as shown in the following figure:

It is seen in this figure that the transverse magnetization decreases in an exponential manner characterized by a time constant, called T2* – also called T2 Star.

In case you're wondering about phase coherence at this stage, a good analogy to think of is that of analogue time clocks in different parts of the world. You may have seen such clocks on international TV news programmes showing the time in different parts of the world or in foreign exchange banks, for instance. Dublin, Sydney, New York and Mumbai, for example, all have clocks which take 60 minutes for one revolution of the big pointer. Their frequencies are identical, in other words. But they each show times separated by several hours because of their geographic location and daylight saving factors. We can conclude that these clocks are out of phase with each other, even though their frequencies are the same. However, all clocks located in Sydney, for example, would have the same phase and we can say that they are in phase coherence. In a similar manner, phase coherence of proton precessions results during RF excitation in NMR and its the loss of this coherence which happens in transverse relaxation.

So, what do these two time constants, T1 and T2*, mean in terms of our tissue sample?

The protons which generate NMR signals are primarily those in cellular fluids and lipids, i.e. nuclei of hydrogen atoms that are relatively free to move within their environment. Hydrogen protons in tightly-bound environments such as within proteins or DNA, however, usually do not contribute to NMR signals and the same situation exists for those in solid structures such as bone.

We can first of all consider a water molecule moving through its environment within our tissue sample as a result of local chemical and physical forces. The magnetic properties of its two hydrogen protons generate a small magnetic field of about 1 mT and the molecule's motion is therefore also influenced by the magnetic properties of other water molecules in its vicinity – and it in turn influences their motion. When excited protons are involved following RF excitation, it is interactions with their local environment which cause them to loose their excess energy and return to the lower energy state with the emission of RF radiation. This can be considered to be the origin of the re-establishment of longitudinal magnetization during relaxation. Chemistry calls this phenomenon spin-lattice relaxation – spin referring to the spinning proton and lattice to its local environment.

The rate at which molecules can move within their environment is related to their size and hence small molecules have a low probability for interaction. This is why fluids such as CSF have long T1 values, for instance – see the table below. Medium-sized molecules (e.g. lipids), in contrast, move more slowly, have a greater probability for interaction as a result, and exhibit relatively short T1 values.

The T2* phenomenon results from the compounding effect of imperfections in the external magnetic field and from so-called spin-spin interactions. These latter are interactions where two nearby protons can cause each other to flip so that one changes from anti-parallel to parallel alignment, while the other changes from parallel to anti-parallel, i.e. one gains the excitation energy from the other. Phase coherence with other excited protons is lost during this exchange and the end result is a relaxation of the transverse magnetization. This spin-spin interaction is also called T2 relaxation. It tends to happen much more rapidly than T1 relaxation and T2 values are therefore generally less than T1 values, as shown in the following table:

T1 and T2 values at 1.5 Tesla.
Tissue	T1 (ms)	T2 (ms)
Muscle	870	47
Liver	490	43
Kidney	650	58
Grey Matter	920	100
White Matter	790	92
Lung	830	80
CSF	2,400	160

Since T2 arises mainly from neighbouring protons, a higher interaction probability exists with larger than with smaller molecules. Macromolecular environments will therefore display shorter T2 values than water-based fluids, e.g. CSF.

A final point to note is that both T1 and T2 measurements from a small volume of tissue result from the integrated motional effects of all molecular species which contain hydrogen protons in that volume, be they those of small molecules, lipids or macromolecules.

#top

Inversion Recovery

A practical problem arises when we try to measure T1 because M_z exists within the Z-axis of the patient. If we could flip the longitudinal magnetization vector into the XY plane, then T1 could be measured using external coils. This is what the Inversion Recovery imaging technique is designed to do.

It involves a two stage excitation process, where an RF pulse of sufficient duration to excite all the lower energy protons is applied initially and this is followed by a second RF pulse after a time interval of duration sufficient for similar numbers of protons to exist in the two alignments. The first stage is illustrated in the following figure:

Illustration of the application of a 180^o RF pulse.

The RF pulse in this case is referred to as a 180^o pulse since the longitudinal magnetization, M_z, has been inverted during the excitation process.

Then a short time interval is used to allow some longitudinal relaxation to occur, as illustrated in the following figure:

Recovery of M_z following a 180^o RF pulse in cases where T1 is long (left panel) and short (right panel).

Notice that M_z recovers relatively slowly when the T1 of the tissue in question is relatively long, and that it recovers quite quickly when T1 is short. We can conclude that the extent of the recovery is related to T1.

A 90^o RF pulse is then used to move M_z into the XY plane so that its amplitude can be measured, as shown in the next figure:

The recovered M_z is moved onto the XY plane using a 90^o RF pulse.

The sequence of RF pulses is shown in the left panel of the figure below, separated by the time interval, TI, and the resultant Free Induction Decay (FID) is shown below it. The amplitude of the FID is dependent on the relative values of T1 and the time interval, TI. For instance, when the time interval is less than T1, the longitudinal magnetization can still be negative when the 90^o is applied – as we saw in an earlier figure. At the other extreme, when the time interval is much greater than T1, the longitudinal magnetization has sufficient time to fully recover and the amplitude of the FID is dependent primarily on the proton density.

The figure in the right panel below shows the dependence of the longitudinal magnetization measured from the FIDs of grey matter and CSF against the time interval, TI. It is seen that both substances generate a negative value of M_z when the time interval is short, as we might expect. It is also seen that M_z increases in each case when the time interval is increased and a region is reached where the values are strongly dependent on T1. It is further seen that a large difference can exist between the measured values for our two substances in this region. This feature gives rise to the generation of contrast between these two substances in T1-weighted images. Typically a time interval of 400 ms is used for such tissue discrimination.

The inversion recovery pulse sequence and resultant FID (left) and the dependence of M_z on the time interval, TI (right).

A final point to note is the proton density-weighting that results when long time intervals are used, with little tissue discrimination. Typically an interval of 700 ms is used for such studies.

There are numerous other pulse sequences used besides Inversion Recovery in MRI. These include:

Spin Echo
Saturation Recovery
Short TI IR (STIR)
Echo Planar Imaging
Small Angle Gradient Echo (SAGE).

The Spin Echo sequence, for instance, can be used to generate a T2-weighting in images. You'll remember from earlier that an FID decays exponentially with a time constant, T2*. This parameter results from the combined effect of two phenomena:

static and oscillating magnetic fields induced by neighbouring protons (and unpaired electrons!) which we've discussed earlier and which generate the T2 information;

small imperfections in the primary magnetic field, B_o, which are referred to as field inhomogeneities, and generate a time constant, T_inh.

These two time constants can be considered to add, such that:

{\frac {1}{T2^{*}}}={\frac {1}{T2}}+{\frac {1}{T_{inh}}}

and the function of the Spin Echo pulse sequence is to disentangle this relationship to extract the T2 information. Note that this pulse sequence can also be used to generate images with a T1 or a proton density weighting depending on various time intervals used in its pulse sequence.

The further treatment of Spin Echo and other pulse sequences is beyond the introductory level of this chapter and you are referred to the Bibliography for additional learning resources. However, you're likely to find that the treatment above will provide a sufficient foundation for developing your understanding of these other pulse sequences.

You might also like to check out the superb e-MRI online learning resource

#top

Spatial Localization of NMR Signals

Since we've seen above how contrast between tissues can be generated in MRI we're now in a position to consider how images are formed. In other words, we'll consider how to localize the origin of the FIDs generated within the patient following RF excitation.

Its helpful to consider an analogy at this stage because this imaging situation is distinctly different to that encountered in nuclear medicine, radiography or sonography where the radiation travels in straight lines and imaging techniques based on emission, transmission and reflection can be readily conceptualized. Its a little bit more difficult in MRI because the radiation is emitted in all directions simultaneously, so that there's little or no chance in knowing its origin from an FID measurement. In other words, the FID only allows the amplitude, frequency and phase of the radio waves to be measured.

A grand piano with a keyboard and scales overlaid.

The analogy we'll use is that of a piano keyboard and the playing thereof. Let's imagine a concert pianist, for instance, on stage and playing the keyboard of a grand piano. Notice that the pianist's fingers hit keys to the left of the keyboard in order to produce a relatively low frequency (i.e. low pitch) sound, keys on the right for high frequency notes and keys in the middle for intermediate frequencies. So, besides hearing the music produced while the pianist plays, we also receive information about where in space the pianist's fingers were located at any individual moment. In technical jargon we can say that we are using a method of spatial localization through frequency discrimination of audio signals to determine locations along the piano's keyboard. This same approach can be applied in MRI using frequency discrimination techniques for the spatial localization of radio signals.

The 3D situation is of course a bit more complex than the 1D piano keyboard, but the principle is basically the same. Frequency discrimination can be achieved by arranging for RF emissions from the patient to have slightly different frequencies depending on their origin. Furthermore, phase discrimination can also be applied so that the phase of the RF emissions can be related to locations within the patient. The fidelity of the frequency and phase discrimination should of course be sufficient so that the corresponding voxels within the patient are sufficiently small enough for clinically-useful views of the anatomy to be generated. Afterall, the fidelity of our frequency discrimination in the case of a piano keyboard is limited at an extreme by the width of the keys!

The magnetic field of the magnet in an MRI scanner is therefore supplemented by small fields introduced using gradient coils so that a linear gradient, for instance, in the field can be established. With such a gradient applied along the patient's Z-axis, we can expect the resonant frequency of hydrogen protons to be different for planes along this axis, as illustrated in the following figure:

Illustration of the application of a linear gradient (in blue) in the magnetic field along a patient's Z-axis so that the resonant frequency can be related to planes along this axis.

Should we wish to excite a single slice along this axis, for instance, all we need to do is generate radio waves of the relevant frequency, as dictated by our earlier equation:

f = γ B_o.

This approach is called slice selective excitation. Both the strength of the gradient field and the bandwidth of the RF pulse produced by the transmitter can influence the thickness of the selected slice as indicated in the following figure:

Effect of gradient field (left) and bandwidth (right) on slice thickness.

We can see in the left panel above that increasing the gradient in the magnetic field from G_a to G_b, for instance, decreases the slice thickness, ΔZ, when the bandwidth, Δf, is kept constant. In comparison, we can see in the right panel that increasing the bandwidth of the RF pulse generated by the transmitter circuitry, from Δf_a to Δf_b for instance, increases the slice thickness.

It is important to note that the slice selection gradient is applied during the application of the RF excitation pulses. The protons in any given plane can then be made to resonate by changing the RF pulse centre frequency, f_o, during the scan.

The G_z gradient and the RF bandwidth control the slice thickness and position in this manner. Note that although the slice selection gradient has been described here in terms of the Z-axis, there is no reason in principle why any other directions cannot be chosen for the application of the gradient. The slice selection is therefore not governed by mechanical considerations, as is the case in X-ray CT, SPECT and PET.

One method of tomographic imaging based on this approach is considered next. Let's assume for simplicity that our patient can be represented by an elongated cube, as shown in the following figure:

Illustration of slice selection during the RF excitation phase using a longitudinal magnetic field gradient.

Notice that our patient is placed in a magnet with a longitudinal gradient in the field, as illustrated by the blue line. The field strength is relatively low, as a result, towards the left of our cube and relatively high towards the right. A central slice of the cube can be excited when an RF pulse of the appropriate frequency is applied. Suppose the selected slice contains two features which generate FIDs, as indicated by the red and green objects.

The RF pulse is used to excite protons in this slice so that they all precess at the same frequency. A transverse gradient in the magnetic field is then applied during the emission phase, as illustrated in our next figure, so that the field strength is low, for instance, to the left of the selected slice and higher to the right of the slice. Precessional frequencies will therefore increase, decrease or remain the same depending on their horizontal location across the slice. The emitted FIDs will therefore contain a range of frequencies which represent this horizontal location.

Illustration of the application of a transverse gradient during the RF emission phase.

The frequency content of the FIDs can be unscrambled by computing the one-dimensional Fourier transform, as illustrated in the figure above, to derive the horizontal spatial locations of the emitters. The resultant Fourier spectrum constitutes one projection, somewhat similar to that acquired in X-ray computed tomography. The subsequent steps are to generate projections at different angles around the slice by repeating steps 1 and 2 in the above figure using different transverse gradients. A series of projections can then result which can be processed using Filtered Back Projection to form an image of the axial slice.

This method is rather slow for clinical application and numerous other methods have been devised. We've considered it here simply because its a good exercise in integrating many of the features that you've learnt about in this chapter and because of its relative simplicity. Faster image acquisition times can be generated using the so-called Spin Warp method, for example. Here, a two-dimensional Fourier transform is applied using repeated slice selective excitations along with frequency-encoding gradients (as we've just described) as well as phase-encoding gradients during the emission phases.

#top

Back to the Nucleus

There are many other features of MRI that are beyond the scope of this chapter. Issues such as safety in magnetic environments are of vital importance and features such as magnetic resonance angiography (MRA), magnetic resonance spectroscopy (MRS), Diffusion Tensor Imaging (DTI), functional MRI (fMRI), contrast media, image artefacts as well as magnet design, shielding and siting are fascinating to consider from a nuclear medicine perspective.

We'll conclude this chapter with an important basic feature which we didn't consider earlier in the interests of clarity. This brings us back to the nucleus and the topic of nuclear spin. You'll appreciate that its not just the hydrogen proton that demonstrates the NMR phenomenon and that it can occur, at the least, in situations where the nucleus has an odd number of protons. In other words, situations where there's an isolated proton which does not have a partner to pair with magnetically in its nucleus.

There's actually three types of nucleus where nuclear spin is concerned – those with:

Odd Mass Number, i.e. nuclei with one unpaired nucleon;

Even Mass Number & Even Atomic Number, i.e. nuclei with no unpaired nucleons;

Even Mass Number & Odd Atomic Number, i.e. nuclei with two or more unpaired nucleons.

Examples of nuclei with a net nuclear spin are given in the following table:

Nucleus	Gyromagnetic Ratio, γ (10⁶rad/s/T)	f_o at 1 Telsa (MHz)
¹H	268	42.58
²H	41	6.53
¹³C	67	10.66
¹⁴N	19	3.02
¹⁹F	253	40.27
²³Na	71	11.3
³¹P	108	17.19